WORK, POWER, AND ENERGY NOTES
Work done is
defined as the product of the distance traveled by a body when force is applied
in any direction except perpendicular to force.
Work done by a constant force is defined as the displacement produced by a constant force when it acts on a body
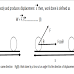
If θ is the
angle between F and s as shown in figure (B), then from equation(I) we have
W = F. s.cosθ …………(II)
If displacement
and the force is in the same direction as shown in figure(I), then θ = 0, cosθ
= 1,
W
= F. s. 1
=F. s
Units of
works
1J = 1N * 1m =
105 dyne * 100cm = 107 erg
Work done by Variable force
Let a variable force is acting on a body to displace it from A to B in a fixed direction. We can consider the entire displacement from A to B is made up of a large number of infinitesimal displacements. One such displacement is shown in the figure from P to Q. As the displacement PQ = dx is infinitesimally small, we consider that along with the displacement, force is constant in magnitudes as well as in direction.
Small amount of
work done in moving a body from P to Q is
dW = F * dx = (PS) (PQ) = area of strip PQRS
Total work done
in moving body from A to B is given by,
W
= ∑dW = ∑F. Dx
If the
displacements are allowed to approach zero, then the number f terms in the sum
increase without limit. And sum approaches a definite value equal to the area
under the curve CD as shown in the figure.
Hence, we can
write
W
= lim dx -> 0 ∑f (dx)
= xbʃxa F dx
=xbʃxa area of strip PQRS
=
total area under the curve between F and x-axis from x = xA to
x= xB
W = Area ABCDA
Hence work done
by a variable force is numerically equal to the area under the force curve and
the displacements.
Energy: It is defined as the capacity to do
work.
Kinetic energy: It is the energy possessed by a body due to its motion.
Expression for Kinetic Energy
Let us consider
a block of mass lying on a smooth horizontal surface as shown in the figure. Let a
constant force F be applied to it such that, after traveling a distance s, its
velocity becomes v.
If a be the
acceleration of the body, then
V2 =
u2 + 2as = 0 + 2as
Or,
v2 = 2as
as initial
velocity, u
= 0,
So, as = v2/ 2 …………… (i)
As work done on
the body = force * distance, then
W
= F * s
= m. a. s ………………. (ii)
From equations
(i) and (ii), we have
W
= m * v2/2 [as = v2/ 2]
= ½ mv2 is the
expression for Kinetic Energy of a body
Potential Energy is the
energy possessed by a body due to its position or configuration.
Work-Energy Theorem
Statement: Total work done by a force acting on a body is the
total change in its kinetic energy.
Proof: Suppose a body of mass m is moving on a smooth
horizontal surface with a constant velocity, u. Let a constant force F acts on
the body from point A to B as shown in the figure such that the velocity
increases to v. The work done by the force is
W = F s
Where 's' is the displacement of the body.
From Newton’s
second law of motion,
F
=ma
Then,
work done is given by
W
= ma s
Let the initial
kinetic energy be K.E1 = ½mu2 and final kinetic energy be K.E2 =
½mv2,
Then,
From the
equation of motion v2 = u2 + 2as,
v2 –
u2 = 2as
or,
as = ½ (v2 – u2)
since,
W = mas = m. ½ (v2 – u2)
=
½mv2 –½mu2
=
K.E2 – K.E1
So, the work
done on moving the body from A to B by applying force F is equal to the increase in Kinetic energy of the body.
Again, we can write the above equation as,
W
= ½mv2 –½mu2
½mv2 =
W +½mu2
That is, the
final kinetic energy of the body is increased and it is the sum of the work
done by the body and its initial kinetic energy.
Principle of Conservation of Energy
According to
this principle, the energy of an isolated system is constant. In other words,
"The energy can neither be created nor be destroyed but can be transformed
from one form to another".
Energy conservation for freely falling bodies:
The mechanical energy of a freely falling body is constant.
Prove
Let a body of
mass ‘m’
at point A at a
height of H
from the
ground. Let the body fall from height. Let B be any instant point
between A and C at distance x from A.
Then its height from the ground is (h-x). Let C
be the ground level and its height is 0.
At A,
K.E.
= 0, since the body
is in rest
P.E.
= mg H,
Where H is the distance between the body and the ground
Total
mechanical energy = K.E. + P.E.
=
0 + mg H
=
mgH …… (i)
At B,
Let the
velocity of the body at point B be vb, then
K.E.
= ½ mvb2 ……… (i)
From the
equation of motion,
V2 =
u2 + 2as
vb2 =
0 + 2gx, where ‘g’ is the acceleration due to gravity and x is the distance traveled by the body from A to B
vb2 =
2gx
In equation
(i),
K.E.
= ½ mvb2
= ½ m. 2gx
= mgx
P.E.
= mg (H-x)
= mgH - mgx
Total
mechanical energy = K.E. + P.E.
= mgx + mgH – mgx
= mgH ..…. (ii)
At point
C,
K.E.
= ½ mvc2 ……. (ii), where vc is the
velocity at point C
From the
equation of motion,
V2 =
u2 + 2as
vc2=
0 + 2gH
vc2 =
2gH
K.E.
= ½ m. 2gH
K.E.
= mgH
P.E.
= mgH = 0
Total
mechanical energy = K.E + P.E.
= mgH ……. (iii)
From this, we
can conclude that the total mechanical energy remains the same at all the points
during the journey since equations (i), (ii), and (iii) are equal.
Conservative and
non-conservative forces
A force is said
to be conservative if the work done by or against the force in
moving the body depends upon only the initial and final positions of the body i.e.
the distance between those bodies. If the work is done by the body while bringing
it into a round circle at the same point, then the force applied to it is called
conservative force.
A force is said
to be non-conservative if work done by or against the force on
a moving body from one position to another depends upon the path followed by
the body.
Power
Power is
defined as the rate at which the work is done.
Mathematically, Power = Work Done/ time
Thus, the power of an agent measures how fast it can do the work.
For constant force,
Power, p = W/t = F.s / t = F. v
Where v = s/t, is linear velocity
If θ be
the angle between F and V, then
P = F. v cosθ
Collisions
Collision is
the mutual interaction between two particles for a short interval of time so
that their momentum and kinetic energy may change. In general, a collision is an
isolated event in which the colliding bodies exert relatively strong forces to
one another for a relatively short time.
There are two types of collisions.
i. Elastic collision: Elastic
collision is the mutual interaction between two bodies where their momentum and
kinetic energy is conserved. It occurs when conservative force is applied to a
body.
Characteristics
of an Elastic collision;
- The momentum is conserved
- Kinetic energy is conserved
- The total energy is conserved
- The forces involved during the interaction are
conservative in nature.
- Mechanical energy is not transformed into any
other form of energy.
Elastic collision in one dimension
If the
colliding bodies move in the same path even after collision then it is said to
be a collision in one direction.
Let us
consider two bodies A and B with masses m1 and m2 moving in a straight line with velocity u1 and u2 such
that u1>u2. After some time, they collide with each other.
Let v1 and v2 be the velocities of the bodies A and B respectively after collision such that v1<v2.
From the
principle of conservation of momentum,
m1u1 + m2u2 =
m1v1 + m2v2 ………….
(i)
m1(u1-v1) = m2(v2-u2)
………………. (ii)
In elastic
collision,
K.E before collision = K.E. after collision
½ m1u12 + ½ m2u22 =
½ m1v12 + ½ m2v22
Or, m1(u1 – v1)(u1+v1)
= m2(u2 – v2)(u2+v2)
……(iii)
Dividing (iii)
by (ii), we get
u1 -u2=v2 -v1 ……….
(iv)
This shows that
in an elastic collision between two particles, the relative velocity of
separation after the collision is equal to the relative velocity of an approach
before the collision.
ii. Inelastic collision
The collision
in which the momentum is conserved but kinetic energy is not conserved is
called Inelastic collision.
Characteristics
of inelastic collision
- The momentum is conserved
- The total energy is conserved.
- Kinetic energy is not conserved.
- Forces involved during the interaction re
non-conservative forces.
- Mechanical energy is transformed into any other
form of energy.
Inelastic
collision in one dimension
Let us consider
two perfectly inelastic bodies of mass m1 and m2. Body A is moving with velocity u1 and B is at rest. After some time, they collide and
move together with the same velocity v. So,
initial momentum before collision = m1u1.
Final momentum
before collision = m1u1
Final momentum
after collision = (m1 + m2) v
Since momentum
is conserved, i.e. (m1 + m2) v =m1u1
V = m1u1/(m1 +
m2) ……. (i)
(K.E
before collision) / (K.E. after collision) = (½ m1u12)
/ ½ (m1 + m2) v2
= m1u12 / (m1 +
m2) [m1u1/ (m1 + m2)]2
= (m1+m2)/m1> 1
Therefore, K.E. before
collision = K.E. after collision